CBSE Sample Papers for Class 12 Applied Mathematics Set 3 with Solutions

Practicing the CBSE Sample Papers for Class 12 Applied Mathematics Set 3 allows you to get rid of exam fear and be confident to appear for the exam.

CBSE Sample Papers for Class 12 Applied Mathematics Set 3 with Solutions

Time Allowed: 3 hours
Maximum Marks: 80

General Instructions:

1. This question paper contains five sections A, B, C, D and E. Each section is compulsory.
2. Section – A carries 20 marks weightage, Section – B carries 10 marks weightage, Section – C carries 18 marks weightage, Section – D carries 20 marks weightage and Section – E carries 3 case-based with total weightage of 12 marks.
3. Section -A: It comprises of 20 MCQs of 1 mark each.
4. Section – B: It comprises of 5 VSA type questions of 2 marks each.
5. Section – C: It comprises of 6 SA type of questions of 3 marks each.
6. Section – D: It comprises of 4 LA type of questions of 5 marks each.
7. Section – E: It has 3 case studies. Each case study comprises of 3 case-based questions, where 2 VSA type questions are of 1 mark each and 1 SA type question is of 2 marks. Internal choice is provided in 2 marks question in each case-study.
8. Internal choice is provided in 2 questions in Section – B, 2 questions in Section – C, 2 questions in Section – D. You have to attempt only one of the alternatives in all such questions.

Section – A
All questions are compulsory. No internal choice is provided in this section

Question 1.
If x ≡ – 4 (mod 3), then a solution for xis: [1]
(A) – 2
(B) 12
(C) 19
(D) 35
Answer:
(D) 35

Explanation: Since 3 |(x + 4)| is true for x = 35

Question 2.
Let m ∈ Z⁺ consider the relation R_m defined as a R_m, b iff a ≡ b (mod rn), then R_m is: [1]
(A) reflexive but not symmetric
(B) symmetric but not transitive
(C) reflexive, symmetric but not transitive
(D) an equivalence relation
Answer:
(D) an equivalence relation

Explanation: The relation R_m defined as a ≡ b (mod m) is reflexive, symmetric and transitive.
∴ R_m is an equivalent relation.

Question 3.
If a ≡ b(mod n) and b ≡ c(mod n), then [1]
(A) a ≡ a(mod n)
(B) b ≡ c(mod a)
(C) a ≡ c(mod n)
(D) None of these
Answer:
(C) a ≡ c(mod n)

Explanation: If a ≡ b(mod n) and b ≡ c(mod n), then n | (b – a) and n | (c – b). Using the linear combination, we get
n | (b – a + c – b) or n | (c – a)
Thus, a ≡ c(mod n)

Question 4.
Assume the current time is 2: 00 p.m. what time (in a,m. or p.m.) will after 65 hours? [1]
(A) 7 am.
(B) 7 p.m.
(C) 3 a.m.
(D) 3 p.m.
Answer:
(A) 7 am.

Explanation: We know that time repeats after every 24 hours
So, we find 65 (mod 24)
∵ 65 = 24 x 2 + 17
So, 65 ≡ 17 (mod 24)
∴ 65 hours is equivalent to 17 hours.
Now, 2 : 00 p.m. + 17 hours = 7: 00 a.m.

Question 5.
Which of the given values of x and y make the following pair of matrices equal? [1]

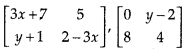
(A) x = \(\frac { -1 }{ 3 }\), y = 7
(B) Not possible to find
(C) y = 7, x = \(\frac { -2 }{ 3 }\)
(D) x = \(\frac { -1 }{ 3 }\), y = \(\frac { -2 }{ 3 }\)
Answer:
(B) Not possible to find

Explanation: It is given that

Equating the corresponding elements, we get:
3x + 7 = 0
⇒ x = – \(\frac { 7 }{ 3 }\)
5 = y – 2
⇒ y = 7
y + 1 = 8
⇒ y = 7
2 – 3x = 4
⇒ x = – \(\frac { 2 }{ 3 }\)
We find that on comparing the corresponding elements of the two matrices, we get two different values of x, which is not possible. Hence, it is not possible to find the values of x and y for which the given matrices are equal.

Question 6.
The number of all possible matrices of order 3 x 3 with each entry O or lis: [1]
(A) 27
(8) 18
(C) 81
(D) 512
Answer:
(D) 512

Explanation: The given matrix of the order 3 x 3 has 9 elements and each of these elements can be either 0 or 1.
Now, each of the 9 elements can be filled in two possible ways.
Therefore, by the multiplication principle, the required number of possible matrices is 2⁹ = 512.

Question 7.

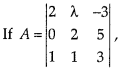
then A^-1 exist, if
(A) λ = 2
(B) λ ≠ 2
(C) λ ≠ – 2
(D) None of these
Answer:
(D) None of these

Explaiation: Given that,

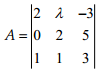
Expanding along R₁,
|A| = 2(6 – 5) – 2( – 5) – 3( – 2)
= 2 + 5λ + 6
We know that A’ exists, if A is non-singular matrix,
i.e., |A| ≠ 0
∴ 2 + 5λ + 6 ≠ 0
5λ ≠ – 8
∴ λ ≠ \(\frac { – 8 }{ 5 }\)
So, A^-1 exists if and only if λ ≠ \(\frac { – 8 }{ 5 }\)

Question 8.
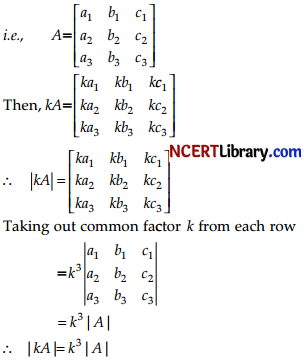
Let A be a square matrix of order 3 x 3, then |kA| is equal to [1]
(A) k|A|
(B) k²|A|
(C) k³|A|
(D) 3k|A|
Answer:
(C) k³|A|

Explanation: We know that, A be a square matrix of order 3 x 3

Question 9.
y = x (x – 3)² decreases for the values of x given by: [1]
(A) 1 < x < 3
(B) x < 0 (C) x > 0
(D) 0 < x < \(\frac { 3 }{ 2 }\)
Answer:
(A) 1 < x < 3

Explanation: Given that,
y = x(x – 3)²
4 = x . 2(x – 3).1 + (x – 3)².1
= 2x² – 6x + x² + 9 – 6x
= 3x² – 12x + 9
= 3(x² – 3x – x + 3)
= 3(x – 3)(x – 1)

So, y = x(x – 3)² decreases for (1,3).
[Since, y’ < 0 for all x ∈ (1, 3), hence y is decreasing on (1, 3)].

Question 10.
If x is real, the minimum value of x² – 8x + 17 is [1]
(A) – 1
(B) 0
(C) 1
(D) 2
Answer:
(C) 1

Explanation: Let,
f(x) = x² – 8x + 17
On differentiating with respect to x, we get
f’(x) = 2x – 8
So,f’(x) = o
= 2x – 8 = 0
2x = 8
∴ x = 4
Now, Again on differentiating w.r.t. x, we get
f”(x)= 2 > 0,∀ x
So, x = 4 is the point of local minima.
Minimum value of f(x) at x = 4
f(4) = 4 x 4 – 8 x 4 + 17 = 1

Question 11.
The order and degree (if defined) of differential equation (1 + \(\frac { dy }{ dx }\))² = (\(\frac{d^2 y}{d x^2}\) )² are: [1]
(A) 3, 2
(B) 2, 2
(C) 3, 3
(D) Not defined
Answer:
(B) 2, 2

Explanation: The highest order derivative present is \(\frac{d^2 y}{d x^2}\) and it is raised to power 2. So, its order is 2 and degree is also 2.

Question 12.
For larger values of ‘n’, Binomial Distribution…………… [1]
(A) loses its discreteness
(B) tends to Poisson’s Distribution
(C) Stays as it is
(D) gives oscillatory values
Answer:
(B) tends to Poisson’s Distribution

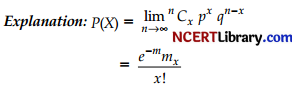
where m = np is the mean of Poisson Distribution.

Question 13.
If ‘m’ is the mean of Poisson Distribution, the standard deviation is given by [1]
(A) \(\sqrt{m}\)
(B) m²
(C) m
(D) \(\frac { m }{ 2 }\)
Answer:
(A) \(\sqrt{m}\)

Explanation: Standard deviation

= \(\sqrt{m}\)
(∵ For Poisson Distribution mean = Variance)

Question 14.
The shape of the Normal curve is……….. [1]
(A) Bell shaped
(B) Flat
(C) Circular
(D) Spiked
Answer:
(A) Bell shaped

Explanation: Due to the nature of the probability mass function, a bell shaped curve is obtained.

Question 15.
The graph of time series is called: [1]
(A) Histogram
(B) Straight line
(C) Historigram
(D) Ogive
Answer:
(B) Straight line

Question 16.
An annuity in which the periodic payment begins on fixed date and continue forever is called: [1]
(A) Sinking fund
(B) Perpetuity
(C) Bond
(D) None of these
Answer:
(B) Perpetuity

Explanation: A perpetuity is a type of annuity that lasts forever, into perpetuity. The stream of cash flows continues for an infinite amount of time.

Question 17.
The reduction of the original price of fixed asset over a period of time due to use is called: [1]
(A) Appreciation
(B) Depreciation
(C) Amortized
(D) None of these
Answer:
(B) Depreciation

Explanation: The monetary value of an asset decreases over time due to use, wear and tear or obsolescence. This decrease is measured as depreciation.

Question 18.
The value of a machine purchased two years ago, depreciates at the annual rate of 10%. If its present value is ₹ 97,200, then its value after 3 years is: [1]
(A) ₹ 70,859 approx
(B) ₹ 71,895 approx
(C) ₹ 80,859 approx
(D) ₹ 88,509 approx
Answer:
(A) ₹ 70,859 approx

Explanation: Given, P = ₹ 97,200, i = 10% p.a.
i = \(\frac { 10 }{ 100 }\) = 0.1
So, value after 3 years
= 97,200 x (1 – 0.1)³
= 97,200 x 0.729
= ₹ 70,858.80
= ₹ 70,859 (approx.)

DIRECTION: For questions 19 and 20, two statentents are given – one labelled Assertion (A) and the other labelled
Reason (R). Select the correct answer to these questions from the codes (A), (B), (C) and (D) as given below:
(A) Both assertion (A) and reason (R) are true and reason (R) is the correct explanation of assertion (A).
(B) Both assertion (A) and reason (R) are true and reason (R) is not the correct explanation of assertion (A).
(C) Assertion (A) is thie but reason (R) is false.
(D) Assertion (A) is false but reason (R) is true.

Question 19.
Assertion (A): The total cost function is given by C(x) = x² + 30x + 1500. The marginal cost when 10 units are produced is ₹ 50.
Reason (R): Marginal cost, M.C. = C’(x) [1]
Answer:
Option (A) is corred

Explanation:
C(x) = x² + 30x + 1500
M.C. = C’(x)
= 2x + 30
M.C. when 10 units are produced
= C’(10)
= ₹ 50

Question 20.
Assertion (A): Mr. Roy purchased a home for ₹ 2,00,000 in 2012. In the next few years, homes in neighbourhood have been selling well due to the new shopping plaza a couple of miles away, which increased the market value of his home. So in 2017, he decided to downsize and sell his home, Based on the current market value during this time, he was able to sell his home for ₹ 2,50,000. The rate of return is 25%. [1]

Answer:
Option (C) is correct

Given, Current value = 2,50,000
Original value = 2,00,000
Rate of return

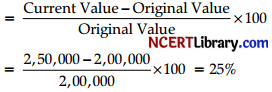
Section – B
All questions are compulsory. In case of internal choice, attempt any one question only

Question 21.
Show that AA and AA’ are both symnwtric matrices for any matrix A.
OR
Without expanding at any stage, find the value of the determinant: [2]

Answer:
Let P = A’ A
P’ = (A’A)’
= A'(A’)’ [ ∵ (AB)’ = BA’]
= A’A = P
So, A’A is symmetric matrix for any matrix A.
Similarly,
Let Q = AA’
Q’ – (AA’)’
= (A’)’A’
= AA’
So, AA’ is symmetric matrix for any matrix A.

OR

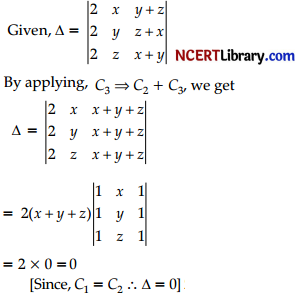
Question 22.
Evaluate:∫\(\frac{d x}{5-8 x-x^2}\)
OR
What is the area bounded by the curve y = log x, X – axis and the ordinates x = 1, x = 2? [2]
Answer:

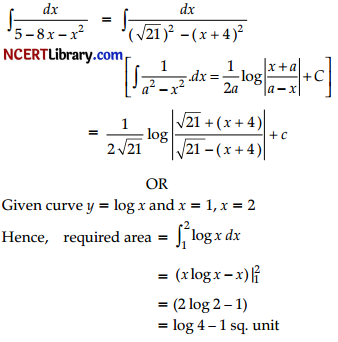
Question 23.
Find the mean E(x) of the numbers obtained on throwing a die having written 1 on three faces, 2 on two faces and 5 on one face. [2]
Answer:
Total no. of observations = 6
∴ P (X = 1) = \(\frac { 3 }{ 6 }\) = \(\frac { 1 }{ 2 }\)
P (X = 2) = \(\frac { 2 }{ 6 }\) = \(\frac { 1 }{ 3 }\)
P (X = 5) = \(\frac { 1 }{ 6 }\)
(where X be a random variable representing a number on die)
∴ Mean, E(X) = ∑p_iX_i
= \(\frac { 1 }{ 2 }\) x 1 + \(\frac { 1 }{ 3 }\) x 2 + \(\frac { 1 }{ 6 }\) x 5
= \(\frac { 1 }{ 2 }\) + \(\frac { 2 }{ 3 }\) + \(\frac { 5 }{ 6 }\)
= \(\frac { 3+4+5 }{ 6 }\)
= \(\frac { 12 }{ 6 }\) = 2

Question 24.
(a) What is population ?
(b) What is sample? [2]
Answer:
(a) A population is a set of similar items or events which is of interest for some question or experiment. A population can be specific or vague. Examples of population defined vaguely include the number of newborn babies in Uttar Pradesh, a total number of tech startups in India, the average height of all exam candidates, mean weight of taxpayers in Lucknow etc. Examples of population defined specifically include a number of fans produced in a particular factory, the number of students in a class, the number of boys and girls in a coaching centre etc.

(b) A sample is a set of data collected from a statistical population by a defined procedure. The elements of a sample are called sample \ size or sample points. Samples are collected and statistics are calculated from the samples, so that one can make inferences from the sample to the population.

Question 25.
Explain the method of fitting a straight line. [2]
Answer:
The method of fitting a straight line is as follows:
(i) The straight-line trend is represented by the equation
Y = a + bX ……………….(1)
where, Y is the actual value, X is time, a and b are constants

(ii) The constants ‘a’ and ‘b’ are estimated by solving the following two normal equations
∑Y = na + b∑X ………..(2)
∑XY = a∑X + b∑X² …….(3)
where, n = number of years given in the data.

(iii) By taking the mid-point of the time as the origin, we get
∑X = o

(iv) When ∑X = 0, the two normal equations reduces to
∑Y = na + b(0); a = \(\frac { ∑Y }{ n }\) = \(\bar{Y}\)
∑XY = a(0) + b∑X²; b = \(\frac{\Sigma X Y}{\Sigma X^2}\)
The constant ‘a’ gives the mean of Y and ‘b’ gives the rate of change (slope).

(v) By substituting the values of ‘a’ and ‘b’ in the trend equation (1), we get the Line of Best Fit.

Section – C
All questions are compulsory. In case of internal choice, attempt any one question only

Question 26.
(a) Compute the last digit in the decimal expansion of 400¹⁰⁰.
(b) Is 4¹⁰⁰ divisible by 3? [3]

OR

A man rows a boat 30 km upstream and 44 km down-stream in lo hours. If he rows 40 km upstream and 55 km downstream in 13 hours, then find the rate at which he rows the boat in still water. [3]
Answer:
(a) The last digit is the remainder when divided by 10. Thus we must compute the congruence class of 4¹⁰⁰ (mod 10).
We have 4² = 6 (mod 10),
and then 6² – 6 (mod 10).
This shows that 4¹⁰⁰ = (4²)⁵⁰ = 6⁵⁰ = 6 (mod 10), so the unit’s digit of 4¹⁰⁰ is 6.

(b) No, since 4¹⁰⁰ = 1¹⁰⁰ = 1 (mod 3). Or we can write 2²⁰⁰ as the prime factorization, and then gcd (3, 2²⁰⁰) = 1.

OR

Suppose the man rows the boat in still water at u km/h, and the rate of water current is υ km/h
Then,
Speed downstream = (u + υ) km/h
Speed upstream = (u – υ) km/h
It is given that he rows the boat 30 km upstream and 44 km downstream in 10 hours.
∴\(\frac { 30 }{ u – υ }\) + \(\frac { 44 }{ u + υ }\) = 10
\(\frac { 30 }{ x }\) + \(\frac { 44 }{ y }\) = 10,
where, u – υ = x
and u + υ = y ……….(i)
He can also row 40 km upstream and 55 km downstream in 13 hours.
∴\(\frac { 40 }{ u – υ }\) + \(\frac { 55 }{ u + υ }\) =13
\(\frac { 40 }{ x }\) + \(\frac { 55 }{ y }\) = 13, …………….(ii)
Multiplying eq.(i) by 4 and eq. (ii) by 3 and subtracting,
we get
\(\frac { 176 }{ y }\) – \(\frac { 165 }{ y }\) = 40 – 39
\(\frac { 11 }{ y }\) = 1
y = 11
u + υ = 11 …………….(iii)
Putting y = 11 in eq.(i), we obtain
\(\frac { 30 }{ x }\) + 4 = 10 ⇒ \(\frac { 30 }{ x }\) ⇒ 6
x = 5
u – υ = 5 ……………..(iv)
Adding eqs. (ii) and (iv), we get
2u = 11 + 5, u = 8
Hence, in still water the man rows the boat at 8 km/h.

Question 27.
In order to promote and increasing the number of students, a coaching centre XYZ announces the scholarship for admission in the coaching centre, students have to clear a I.Q. test conducted by the coaching centre. A random sample of 10 boys had the following I.Qs: 70, 120, 110, 101, 88, 83, 85, 95, 98, 107, 100. [3]

Find the t-statistic for the given data, it titean. I.Q. of the population is considered as 100.
Do these data support the assumption of a population mean I.Q. of 100? [Given t₉(0.05) = 2.262]
Answer:
We have μ = population mean = 100
n = sample size = 10
computation of \(\bar{x}\) and S

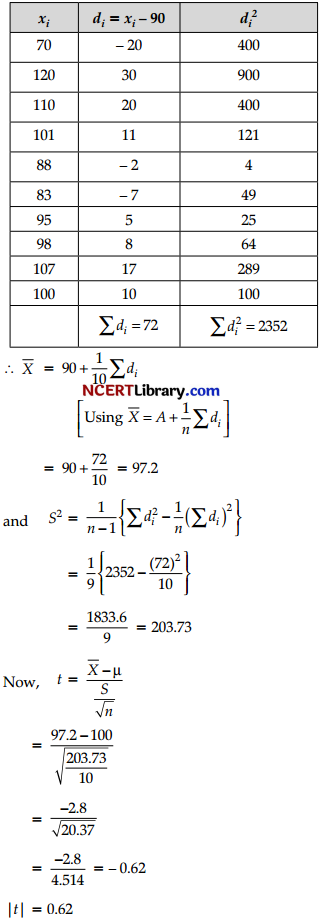
We define,
Null Hypothesis H₀: The data are consistent with the assumption of a mean l.Q. of 1oo in the population.
Alternate Hypothesis H₁: The mean I.Q. of population + 100.

Question 28.
Calculate trend values from the following data assuming 5 – yearly and 7 – yearly moving average. [3]

Year	1	2	3	4	5	6	7	8
Value	110	104	98	105	109	120	115	110
Year	9	1o	11	12	13	14	15	16
Value	114	122	130	127	122	118	130	140

Answer:
Calculation of Trend values by moving average method:

Year	Value	Moving Totals		5 Year	7 Year
		5 Year	7 Year
1	110	–	–	–	–
2	104	–	–	–	–
3	98	526	–	105.2	–
4	105	536	761	107.2	108.71
5	109	547	761	109.4	108.71
6	120	559	771	111.8	110.14
7	115	568	795	113.6	113.57
8	110	581	820	116.2	117.14
9	114	591	838	118.2	119.71
lo	122	603	840	120.6	120.00
11	130	615	843	123.0	120 .43
12	127	619	863	123.8	123.29
13	122	627	889	125.4	127.00
14	118	637	–	127.4	–
15	130	–	–	–	–
16	140	–	–	–	–

Question 29.
Maximise Z = x + 2y
Subed to the constraints
x + 2y ≥ 1oo
2x – y > 0
2x + y ≤ 200
x, y ≤ 0 [3]

OR

Solve the following linear problem graphicaUy.
Maximize Z = 5x + 3y
Subject to constraints:
3x + 5y ≤ 15
5x + 2y ≤ 10
x ≥ 0, and y ≥ 0
Answer:
Z = x + 2y and x + 2y ≥ 100, 2x – y ≤ 0, 2x + y ≤ 200,
x, y ≥ 0

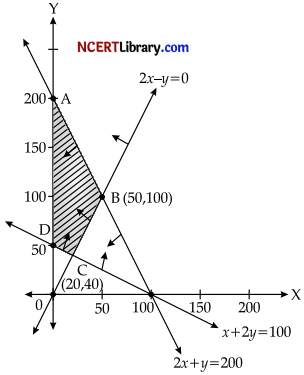
Z(A) = 0 + 400 = 400
Z(B) = 50 + 200 = 250
Z(C) = 20 + 80 = 100
Z(D) = 0 + 100 = 100
∴Max (Z = 400) at x = D, y = 200

OR

Given LPP is:
Maximize Z = 5 x+ 3y
Subject to constraints:
3x + 5y ≤ 15
5x + ≤ lo
x ≤ O, and y ≥ 0

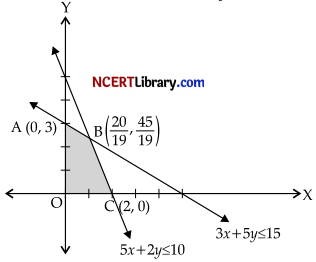
As shown in graph, the shaded bounded region OABCO represents the common region of the above
inequations. This region is the feasible region of the given L.PP.
The co-ordinates of the vertices (corner points) of the
shaded bounded feasible region are 0(0, 0), A(0, 3), B(\(\frac { 20 }{ 19 }\), \(\frac { 45 }{ 19 }\)) and C(2, 0).
These points have been obtained by solving the equations of the corresponding intersecting lines, simultaneously.
The value of the objective function as these points are given in the following table:

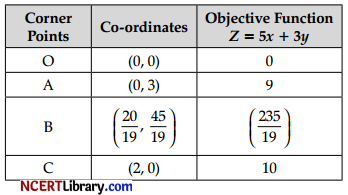
(20 45) Clearly, Z is the maximum at P(\(\frac { 20 }{ 19 }\), \(\frac { 45 }{ 19 }\))
Hence, x = \(\frac { 20 }{ 19 }\), y = \(\frac { 235 }{ 19 }\) is the optimal solution of
the given LPP.
235
The optimal maximum value of Z is \(\frac { 235 }{ 19 }\) when
x = \(\frac { 20 }{ 19 }\) and y = \(\frac { 45 }{ 19 }\)

Question 30.
Calculate the EMI under ‘Flat Rate System’ for a loan of ₹ 5,00,000 with 10% annual interest rate for 5 years. [3]
Answer:
We have, P = Principal = ₹ 5,00,000
i = rate of interest per month
= \(\frac { 10 }{ 12 x 100 }\) = \(\frac { 1 }{ 120 }\)
n = No. of installments
= 12 x 5 = 60
∴ EMI = ₹ P(i + \(\frac { 1 }{ n }\))
= ₹ 5,00,0001 (\(\frac { 1 }{ 120 }\) + \(\frac { 1 }{ 60 }\))
= ₹ 5,oo,ooo (\(\frac { 1+2 }{ 120 }\))
= ₹ 5,oo,ooo (\(\frac { 3 }{ 120 }\))
= \(\frac { ₹5,00,000 }{ 40 }\) = ₹ 12,500

Question 31.
A company establishes sinking fund to provide for the payment of ₹ 1,00,000 debt. maturing in 5 years. Contributions to the fund are to be made at the end of every year. Find the amount of each annual deposit if interest is 15% per annum. Given ((1.15)⁵ = 2.011] [3]
Answer:
L.et each annual deposit to the sinking fund be ₹ P
Since, Sinking Fund, A = P x \(\frac{(1+i)^n-1}{i}\)
1,00,000 = P x \(\frac{(1+0.15)^5-1}{0.15}\)
or, 1,00,000 = P x \(\frac{(1.15)^5-1}{0.15}\)
or, 1,00,000 = P x \(\frac { 2.011-1 }{ 0.15 }\)
or, 1,00,000 = P x \(\frac { 1.011 }{ 0.15 }\)
or, P = \(\frac { 1,00,000 x 0.15 }{ 1.011 }\)
= ₹ 14,836.795

Section – D
All questions are compulsory. In case of internal choice, attempt any one question only

Question 32.

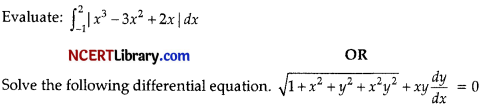
Answer:
The given definite integral

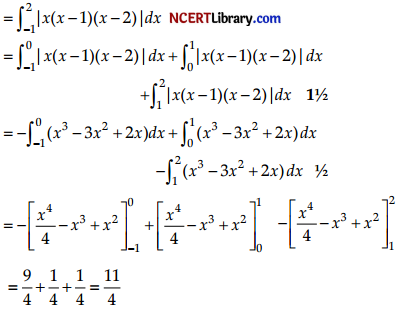
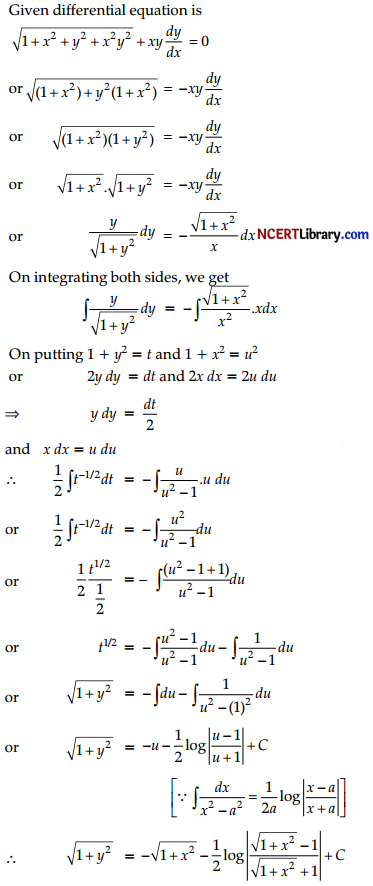
Question 33.
(a) The marks obtained in a certain exam follow normal distribution with mean 45 and SD 10. If 1,300 students appeared at the examination, calculate the number of students scoring (i) less than 35 marks and (ii) more than 65 marks.

(b) 900 light bulbs with a mean life of 125 days are installed in a new factory. Their length of life is normally distributed with a standard deviation of 18 days. What is the expected number of bulbs expire in less than 95 days? [5]

OR

(a) Two cards are drawn successively with replacement from a well shuffled pack of 52 cards. Find the probability distribution of the number of diamond cards drawn. Also, find the mean and the variance of the distribution.

(b) A telephone switch board handles 600 calls on the average during a rush hour. The board can make a maximum of 20 connections per minute. Use poisson distribution to estimate the probability the board will be over taxed during any given minute. [e^-1 = 0.00004539] [5]
Answer:
(a) Let X be the normal variate showing the score of the candidate with mean 45 and standard deviation 10.
(i) less than 35 marks When X = 35
Z = \(\frac { X – μ }{ σ }\) = \(\frac { 35 – 45 }{ 10 }\) = – 1

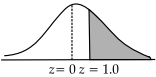
P(X < 35) = P(Z < – 1) P(Z > 1) = 0.5 – P(0 < Z < 1)

OR

(a) Let X = denote the random variable. X = 0, 1,2, n = 2, p = 1/4, q = 3/4

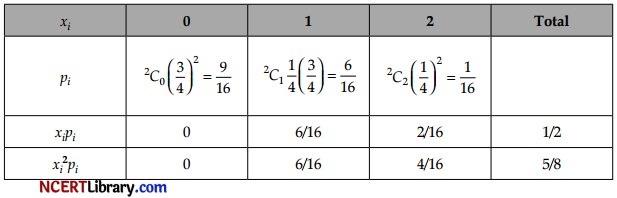
Mean = ∑x_ip_i = \(\frac { 1 }{ 2 }\)
Variance = ∑x_i ²p_i – (∑x_ip_i)²
= \(\frac { 5 }{ 8 }\) – \(\frac { 1 }{ 4 }\) = \(\frac { 3 }{ 8 }\)

(b) Mean (per minute) = \(\frac { 600 }{ 60 }\) = 10
Hence, the probability for using 0 to 20 calls per minute

Thus, the probability that the board will be over taxed during any given minute i.e. when the calls will be more than 20 connections per minute.

Question 34.
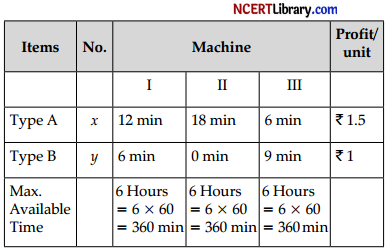
A manufacturer manufactures two types of tea-cups, A and B. Three machines are needed for manufacturing the tea cups. The time in minutes required for manufacturing each cup on the machines is given below:

Type of Cup	Time in minutes
	Machine I	Machine II	Machine III
A	12	18	6
B	6	0	9

Each machine is available for a maximum of six hours per day. If the profit on each cup of type A is ₹ 1.50 and that on each cup of type B is ₹ 1.00, find the number of cups of each type that should be manufacturing in a day to get maximum profit. [5]
Answer:
Let x be the number of A type tea cups and y be the number of B type tea cups.

The problem can be formulated as
Max. Z = 1.50x + 1.00y
i.e., Max. Z = 1.50x + y
Subject to the constraints:
12x + 6y ≤ 360,i.e.,2x + y ≤ 60
18x + 0.y ≤ 360,i.e.,x ≤ 20
6x + 9y ≤ 360,i.e.,2x + 3y ≤ 120
and x ≥ 0, y ≥ 0 (Non-negative constraints)
We draw the straight lines:
2x + y = 60, x = 20, 2x + 3y = 120

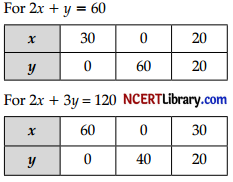
The shaded portion shows the feasible region. The points B and C are points of intersection of lines 2x + y = 60, with 2x + 3y = 120 and x = 20 with 2x + y = 60 respectively.

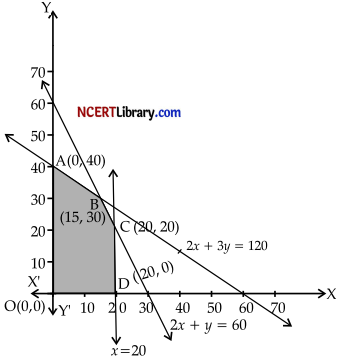
Thus, the 5 corner points of the feasible region are 0(0,0), A(0, 40), B(15, 30), C(20,20) and D(20, 0).
Z = 1.5x + y

Comer Point	Value of Z 1.5x + y
0(0, 0) A(0, 40) B(15, 30) C(20, 20) D(20,0)	o 40 52.5 (Max) 50 30

Clearly, the maximum profit is at x = 15 and y = 30.
Thus, the manufacturer should manufacture 15 cups of type A and 30 cups of type B to get maximum profit inaday.

Question 35.
Mr. Kundan wants to purchase a car for ₹ 25,00,000 with down payment of ₹ 10,00,000. If he can amortize the balance at 8% per annum compounded monthly for 7 years.
(i) What is the monthly payment?
(ii) What is the total interest paid?
Given (1.0066)⁸⁴ = 1.7474 5
Answer:
Amortized Amount i.e., P = Cost of car – Cash down payment
P = ₹ (25,00,000 – 10,00,0(X))
= ₹ 15,00,000
i = \(\frac { 0.08 }{ 12 }\) = ₹ 0.0066
n = 7 x 12 = 84

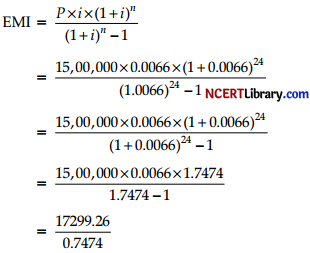
= ₹ 23145.919 ≈ ₹ 23146
Total interest paid = n X EMI – P
= 84 x 23146 – 15,00,000
= 19,44,257 – 15,00,000
= ₹ 4,44,257

Section – E
All questions are compulsory. In case of internal choice, attempt any one question only

CASE STUDY – I

Question 36.
Riya a biologist runs an experiment in the laboratory involving a culture of bacteria. She is doing this experiment first time. She notices that the mass of the bacteria in culture increases exponentially. The bacteria count in the culture is ₹ 1,00,000. The rate of growth of bacteria is proportional to the number of bacteria present in the culture. Also, the number of bacteria is increased by 10% in 2 hours.

Based on the above given information answer the following questions:
(i) If y be the number of bacteria at time t, then find the differential equation that models in the given scenario.
(ii) Find the general solution of the associated differential equation.
(iii) Find the value of constant of integration and bacteria count after 2 hours.

OR

In how many hours will the bacteria count reach 2,00,000?
Answer:
(i) If y be the number of bacteria at time t, then
\(\frac { dy }{ dt }\) ∝ y
\(\frac { dy }{ dt }\) = ky

(ii)
\(\frac { dy }{ dt }\) = ky
\(\frac { dy }{ y }\) = kdt
Integrating both sides, we get
logy = kt + c

(iii) Put t = 0 and y = 1,00,000 in equation log y = kt + c, we get
log 1,00,000 = k x 0 + c
c = log 1,00,000
When, t = 2 hours
y = 1,00,000 + 10% x 1,00,000
= 1,10,000

OR

Put c = log 1,00,000 andy = 1,10,000 in log y = kt + c
we get
log y = kt + log 1,00,000 ……………..(i)
Now when t = 2, y = 1,10,000
So, from equation (i), we get
log 1,10,000 = 2k + log 1,00,000
log(1,00,000 – 1,10,000) = 2k
k = \(\frac { 1 }{ 2 }\) log (\(\frac { 11 }{ 10 }\))
[log m – log n = log – \(\frac { m }{ n }\) ]
Now finding t when bacteria = 2,00,000
log 2,00,000 = \(\frac { 1 }{ 2 }\) log (\(\frac { 11 }{ 10 }\)) t + log (1,00,000)
log (\(\frac { 2,00,000 }{ 1,00,000 }\)) = \(\frac { 1 }{ 2 }\) log (\(\frac { 11 }{ 10 }\)) t
log 2 = \(\frac { 1 }{ 2 }\) log (\(\frac { 11 }{ 10 }\)) t
t = \(\frac{2 \log 2}{\log \left(\frac{11}{10}\right)}\)
= \(\frac { 2 x 0.6931 }{ 0.0953 }\)
= 14.55 years

CASE STUDY – II

Question 37.
Two schools P and Q want to award their selected students on the values of the Discipline, Politeness and Punctuality. The school P wants to award ₹ x each, ₹ y each and ₹ z each for the three respective values to its 3, 2 and 1 students with the total award money of ₹ 1,000. School Q wants spend ₹ 1,500 to award its 4, 1 and 3 students on their respective values (by giving the same award money for the three values as before). The total amount of awards for one prize on each value is ₹ 600.

(i) write the system of equations for the given information. [1]
(ii) If the given system of equations can be written as AX = B, then find |A| : [1]
(iii) Find A^-1. [2]

OR

Find award money for Discipline, Politeness and Punctuality.
Answer:
(i) According to the given conditions of the questions,
we have
3x + 2y + z =1000
4x + y + 3z =1500
x + y + z = 600

(ii) The given system of the questions can be written as
AX= B,i.e.

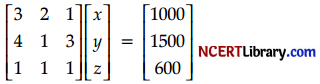
Now, |A| = 3(1 – 3) – 2(4 – 3) + 1(4 – 1)
= – 6 – 2 + 3 = – 5

(iii) Since |A| ≠ 0, thus A^-1 exists, so the given system of
equation has unique solution X = A^-1B

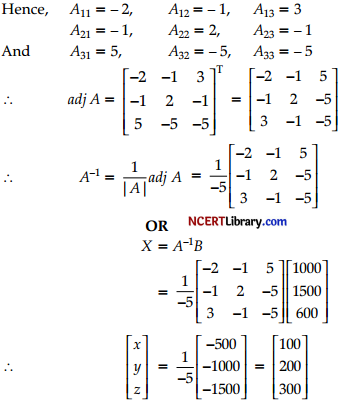
Hence, the award money for Discipline is ₹ 100.
the award money for Politeness is ₹ 200.
the award money for Politeness is ₹ 300.

CASE STUDY- III

Question 38.
A linear inequation in one variable is of the form ax + b < 0 or,ax + b ≤ 0 or ax + b > 0 or, ax + b ≥ 0
We follow the following algorithm to solve a Linear inequation in one variable. [2]
Algorithm:
Step I: Obtain the linear inequation
Step II: Collect all terms involving the variable on one side of the inequation and the constant term on the other side.
Step III: Simplify both sides of inequality in their simplest forms to reduce the inequation in the form ax < b, or ax ≤ b, or ax > b, or ax ≥ b
Step IV: Solve the inequation obtained in step III by dividing both sides of the inequation by the coefficient of the variable.
Step V: Write the solution set obtained in step IV in the form of an interval on the real line.
(i) Solve the linear inequation 2x – 8 ≤ 0. [1]
(ii) Solve the linear inequality – 3x + 15 < 0. [1] (iii) Solve for X: \(\frac { 4 }{ x+1 }\) ≤ 3 ≤ \(\frac { 6 }{ x+1 }\) (x > 0) [2]

OR

Solve for X: – 3 ≤ \(\frac { 2 – 3x }{ 4 }\) ≥ 9
Answer:
(i) We have
2x – 8 ≤ 0
(2x – 8) + 8 ≤ 0 + 8
2x ≤ 8
x ≤ 4

(ii) We have
– 3x + 15 < 0
– 3x < – 15 \(\frac { – 3x }{ 3 }\) > \(\frac { -15 }{ -3 }\)
x > 5

(iii) Consider first two inequalities, \(\frac { 4 }{ x+1 }\) ≤ 3
4 ≤ 3(x + 1)
4 ≤ 3x + 3
4 – 3 ≤ 3x
[subtracting 3 on both sides]
1 ≤ 3x
x ≥ \(\frac { 1 }{ 3 }\) …………(i)
and consider last two inequalities,
3 ≤ \(\frac { 6 }{ x+1 }\)
3(x + 1) ≤ 6
3x + 3 ≤ 6
3x ≤ 6 – 3
[subtracting 3 on both sides]
3x ≤ 3 [dividing by 3]
∴ x ≤ 1
From Eqs.(i) and (ii),
x ∈ [ \(\frac { 1 }{ 3 }\), 1 ]
\(\frac { 1 }{ 3 }\) ≤ x ≤ 1
We have -5 ≤ \(\frac { 2-3x }{ 4 }\) ≤ 9
– 20 ≤ 2 – 3x
[multiplying by 4 on both sides]
3x ≤ 2 + 20
3x ≤ 22
x ≤ \(\frac { 22 }{ 3 }\)
and \(\frac { 2-3x }{ 4 }\) ≤ 9
2 – 3x ≤ 36
– 3x ≤ 36 – 2
– 3x ≤ 34
3x ≥ – 34
x ≥ – \(\frac { – 34 }{ 3 }\)
– \(\frac { 34 }{ 3 }\) ≤ x ≤ \(\frac { 22 }{ 3 }\)
x ∈ [ \(\frac { – 34 }{ 3 }\), \(\frac { 22 }{ 3 }\) ]